China Squares is the twin game of China Octangle and it likewise uses 255 of the 256 square tiles of a two-groups transcendental solution of the Octopuszle as a board.

Material On the left you can see the Octopuszle set. It consists of all possible patterns of zero to eight lines, also called 'beams', radiating from the centre to the edges and corners, in every possible orientation or reflection. Both players have a sufficient number of stones, black for one, white for the other. The puzzle knows compact, transcendental and starmap solutions. A starmap has to our knowledge never even been tried and compact solutions are very hard to achieve. Transcendental solutions of two groups are used as playing boards for China Octangle or its twin China Squares. The single blank is one 'group' and it plays no role in the games. The other one is an orthogonally and/or diagonally connected group of 255 squares. Squares may also be referred to as 'cells'.

A transcendental solution with lines On the left you see the big group of a transcendental solution in two groups, with the lines needed to create one still visible. The single blank square has been omitted.

The same board without lines Here's the same solution with the lines omitted, but since it is transcendental, no information has been lost. Each square is still uniquely determined by the pattern that its orthogonal and diagonal neighbours form around it: the defining characteristic of a transcendental solution. The applet can show only cells, only lines, or both.

Rules

• The game starts on an empty board, White plays first, Black is entitled to a swap.
• If a player on his turn puts one stone on a vacant square, then all vacant squares that are open to further placements in the same turn are highlighted. These are squares with the same pattern of lines and thus neighbours, both orthogonally and diagonally, as the square of the first stone that is placed, including all possible rotations modulo 45 dergees, and reflections.
• Patters arising from 45 degrees rotations are considered the same, like these two  
• Placement is mandatory and a player must place the maximum possible number of stones. This is 9 minus the number of orthogonal and diagonal neighbours of the square on which the first stone is placed.

Obviously only one stone can be placed on the one square with eight eight neighbours. But place the first stone on a square with one neighbour, then you can place eight stones, provided all eight squares with one neighbour are vacant. It will not always be possible to place stones up to the theoretical limit.

Definition
A group consists of a stone and all stones that can be reached from it via single orthogonal or diagonal steps on like coloured stones. A groups is maximal, meaning that part of a group is not a group. Note that since groups do include diagonal connections, black and white groups can be intertwined. Diagonal cross-cuts are entirely possible.

Goal
The game ends when the board is full, or earlier if a player resigns. On a full board the players' respective numbers of stones and groups are compared. A player gets one point for every stone of his colour and loses 'P' points for every separate group, where 'P' is the agreed penalty for the game, which is 2, 4, 6 or 8 points. Single stones are groups by definition. Because the playing area has an odd number of cells the count leads to an inevitable decision.

Strategy
Generally speaking a board has denser areas around the centre, where squares are closely packed together, and more open areas along the periphery, where squares are scattered and farther apart. Since the maximum number of stones a player may place is equal to 9 minus the number of neighbours the square on which the first placement is made, a player gets to play less stones in those dense areas and more stones in the open ones. But in the latter case they are farther apart and thus harder to connect. And with penalty points for every separate group, so are connections. The higher the penalty, the more important connectability becomes.
Unlike its twin game, China Squares is a cold game. In the opening and middle game mandatory maximal placement makes that connections or blocks will often have the creation of new groups as a side-effect. In those phases it is crucial to ensure maximal opportunities for connecting them later on. Towards the endgame placement on cells with fewer neighbours becomes more frequent, and the risks of having to create new groups increases. It is good strategy, therefore, to occupy cells that are adjacent to such low-number-of-neighbours cells, to prevent having to collect penalty points later on if and when they must be occupied.
Every board has the same general structure, but it will also have specific peculiarities that provide a lot of leeway for the general strategy. It's good to take that into account.

Rotational sets that do not include reflections

The set of rotations by 45 degrees of any of these sixteen 3-line squares does not include its reflections.

The set of rotations by 45 degrees of any of these sixteen 3-line squares does not include its reflections.

The set of rotations by 45 degrees of any of these sixteen 4-line squares does not include its reflections.

The set of rotations by 45 degrees of any of these sixteen 4-line squares does not include its reflections.

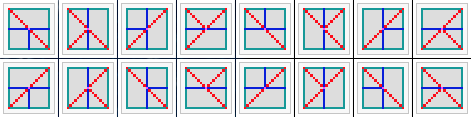
The set of rotations by 45 degrees of any of these sixteen 5-line squares does not include its reflections.

The set of rotations by 45 degrees of any of these sixteen 5-line squares does not include its reflections.

These six sets of 16 squares each represent a strategic focus point. They are in between the dense centre and the open periphery, in between great connectivity and many placements per turn, the middle men in every respect. But there's something special about them because the set of rotations of any of them does not include the set of reflections. So instead of allowing a maximum of seven follow-up placements if the first stone is placed on one of them, they offer a maximum of fifteen stones to choose from. That of course will on average give more and thus better options. Consequently they are much sought after in the opening.


How I invented ... China Squares

Play China Squares interactively


China Octangle © MindSports